Determination of source strength of 241Am in fire alarms
Experimental procedure:- Mount a metal plate-supported 241Am fire alarm source on an aluminum ring covered with transparent tape on both sides.
- Mount the standard source of 241Am on an aluminum ring with adhesive tape only on one side.
- Use the two sources and find a suitable counting distance between source and detector (start with a distance of about 5 cm) so that the counting rate is kept at a reasonable and not too high level,
- Mount the fire alarm source at the decided distance and count the source for 300 s (live-time).
- Store the spectrum in a dedicated folder on the PC.
- Mount the standard source in the same position and count for 300 s (live-time).
- Store the spectrum in the same folder.
- Use the Maestro program and integrate the photo peaks in both spectra to derive at their respective peak areas.
Calculations:
The standard source has a known activity As,i at a certain defined date. Let us denote the time from this date until today with td (decay time, in days). One finds the standard source strength today, As,t, by the formula:
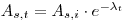 |
(1) |
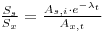 |
(2) |
Solved with respect to Ax,t,we have:
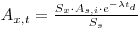 |
(3) |
For the calculations Look up the half-life of 241Am from the nuclide chart and obtain the certified activity of the standard source and the certification date from the lab-assistant.
| Table 1: Data handling and calculations for 241Am |
|||
|---|---|---|---|
| Source |
Recorded number of counts per 300 s, S |
Original decay rate at certification time (Bq) |
Decay rate today (Bq) |
| Standard |
Ss= |
As,i= |
As,t= |
| Fire alarm |
Sx= |
Ax,t= |
|
Determination of concentration of KCl in so-called “health salt” – SELTIN
SELTIN is a popular table salt in Norway. It contains substantial amounts of KCl instead of NaCl. In this section we shall determine the specific activity and the activity concentration of 40K and the fraction of KCl in weight % in SELTIN
| Table 2: Some physical parameters for 40K |
|||||
|---|---|---|---|---|---|
| Molweight of KCl MKCl (g/mol) |
Atomic weight of K MK (g/mol) |
Avogadro’s number NA |
Natural abundance of 40;K YK-40 (%) |
Branching ratio of 1462 keV, Iabs (%) |
Half-life of 40K T1/2 (years) |
| 74.551 |
39.1 |
6.023 1023 1023 |
0.0117 |
10.7 |
1.28 109 109 |
Remember in addition that the following relation is generally valid:
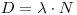 |
Eqn. 4 |
The general relation between total counting efficiency, decay rate and counting rate is:
 |
Eqn. 5 |
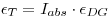 |
Eqn. 6 |
Since the sample weight and geometry are nearly identical for the two samples, we can suppose that the total counting efficiency, εT, is also the same for both samples. Therefore, the decay rate of 40K in SELTIN is calculated by the formula:
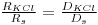 |
Eqn. 7 |
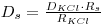 |
eqn. 8 |
Practical Procedures
- Start the spectrometer with a preset live-time of 30 min for recording of background.
- Weigh in a counting sample of KCl (wKCl) in a suitable lid-covered plastic box.
- Weigh a similar sample of SELTIN (wS) in an identical counting box.
- If the background counting has not finished already, stop the spectrometer, note the counting time (live-time) tB and store the spectrum in a dedicated file.
- Mount the KCl-sample, count for a preset time tKCl and store the spectrum in a dedicated file after the spectrometer has stopped.
- Mount the SELTIN sample, count for a preset time tS and store the spectrum in a dedicated file after end of counting.
Spectra Handeling and Calculations
Determine the net area (S±σS) for the 1462 keV gamma peak in the three recorded spectra. Then carry out the calculations to fill into the table below:
| Table 3. Data handling and calculations for 40K |
|
|---|---|
| Operations |
Result of Operations |
| Net area of 1462 keV in background spectrum, SB±σSB |
|
| - Counting time tB |
|
| - Background counting rate, RB±σSB |
|
| Net area of 1462 keV in KCl spectrum, SKCl±σSKCl |
|
| - Counting time tKCl |
|
| - Background-corr. KCl sample counting rate, RKCl±σRKCl |
|
| Net area of 1462 keV in SELTIN spectrum, SS±σSS |
|
| - Counting time tS |
|
| - Background corr. SELTIN sample counting rate, RS±σRS |
|
| Net weight of the KCl-sample, wKCl |
|
| - Weight of K in the KCl sample, wK,KCl |
|
| - Number of K-atoms in the KCl-sample, NK,KCl |
|
| - Number of 40K atoms in the KCl sample, NK-40,KCl |
|
| - Calculated decay rate of 40K in the KCl sample, DK-40,KCl |
|
| - Specific activity of 40K in the KCl sample, As,KCl |
|
| - Activity concentration of 40K in the KCl sample, Ac,KCl |
|
| - Total counting efficiency of 40K in KCl sample,εT,KCl |
|
| - Total counting efficiency of 1462 keV in KCl sample, εDG,KCl |
|
| Net weight of the SELTIN sample, wS |
|
| - Decay rate of 40K in the SELTIN sample, DK-40,S |
|
| - Activity concentration of 40K in the SELTIN sample, Ac,S |
|
| - Number of 40K-atoms in the SELTIN sample, NK-40,S |
|
| - Number of K-atoms in the SELTIN sample, NK,S |
|
| - Weight% of KCl in SELTIN |
|
Gamma Spectroscopy on Thorium and Uranium and Progeny
Procedure:
- Record gamma spectra with the NaI(Tl) detector for the following samples and store the spectra in dedicated files:
a. Uranium oxide of natural uranium isotope composition.
b. Uranium with about 1.5% enrichment in 235U.
c. Uranium with about 20% enrichment in 235U.
d. Th(NO3)2. - Determine the energies of the strongest peaks and find the corresponding radionuclei. Use the attached spectra and tables.
- Plot the spectra and attach the plots to this report with energies and radionuclides indicated on the plot.
- Determine the energy counting efficiency, εDG, for the energies in the range around 600 keV for a certain counting geometry (ask lab supervisor) by using a standard source of 137Cs (661.6 keV).
- Prepare a small piece of native radioactive rock, record its weight and accumulate a gamma spectrum in this counting geometry.
- Plot the spectrum and find the corresponding radionuclei. Use the attached spectra and tables. Attach the plot to this report.
- If the activity in the rock is predominantly from 238U and progeny: Integrate the peak at 609 keV (belonging to 214Bi) and determine the activity concentration of 214Ra in the rock. Find necessary data for Iabs in the attached tables.
- If the activity in the rock is predominantly from 232Th and progeny: Integrate the peak at 583 keV (belonging to 208Tl) and determine the activity concentration of 208Bi in the rock. Find necessary data for Iabs in the attached tables.
- Make a table of data used and the results achieved for the rock sample.
- Prepare a counting sample of scale from the North Sea petroleum operations in a small lid-covered plastic container, and determine the net weight.
- Accumulate a gamma spectrum of the scale sample and determine the energies of the strongest peaks. Attach spectrum.
- Integrate the 609 keV peak of 214Bi and determine the activity concentration of 214Bi in the sample.
- Make a table of data used and the results achieved for the scale sample.
- Perform an energy calibration of a HPGe-semiconductor detector. Let the lab supervisor demonstrate the superior energy resolution of this detector for some of the samples analysed with the NaI(Tl)-detector. Determine the gamma energies and the corresponding nuclei. Attach the plots.
- Compare the HPGe-and NaI(Tl)-spectra: Describe the main differences and indicate advantages and disadvantages with the two detectors for TENORM-containing scale analysis for the petroleum industry.